Lothar Göttsche
International Centre for Theoretical Physics, Trieste
Thursday 21 March 2024, 4.00pm – 5.00pm
Lecture Theatre D (Mathematical Institute)
Curve counting, refined and tropical
This talk deals with moduli spaces in algebraic geometry and enumerative geometry of curves.
An old question in enumerative geometry is to determine the numbers of plane curves of degree d and genus g passing through a suitable number of general points (so that this number is finite), and the corresponding generalization from the plane to a general algebraic surface. One can ask this question both for complex curves (Severi degrees and Gromov-Witten invariants) and real curves (Welschinger invariants).
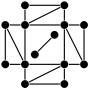
Both of these problems can be reduced to combinatorics using tropical geometry, replacing the curves by a certain class of graphs, the tropical curves, and counting them with suitable integer multiplicities. These tropical curves can be obtained as limits of amoebas, which are obtained by taking the logarithms of the absolute value of the coordinates of the points on the original curves.
We introduce polynomial multiplicities for tropical curves, leading to a polynomial count of curves, interpolating between the real and complex curve counts. We review different interpretations of these polynomial counts by several authors. One of them is counting real curves by the areas of their corresponding amoebas. Another one is that the refined count of genus g curves is a combination of Gromov-Witten invariants counting curves of all higher genera.
series: Pure Mathematics Colloquium
organiser: Scott Harper